True Odds Calculator

The fundamental basis to beat the bookmakers is to always bet over the True Odds.

Do you know them, do you take them into account at the time of betting?

If not, you are a ship without sails no rudder and the day of your capsizing as a bettor is near.

This true odds calculator is all you need to make sure you bet with value.

I’ll leave it here, and just below I’ll explain how to use it and why and when do you need to remove the margin of the bookmakers.

What are the true odds?

As you know, (and if not, you should), the betting odds are related to the probabilities of each outcome.

The lower odds, the higher probability, and vice versa.

To explain the usefulness of this calculator and why it is a MUST in any value betting strategy, let’s start at the beginning.

What are the probabilities?

We can approximate the probabilities with the formula:

In the following football match between “Real Madrid” and “Atlético de Madrid” (odds taken saturday 11th, 17:16):

• Real Madrid to win = 1/ 2.09= 0.478
• Draw = 1/ 3.59 =0.279
• Atlético de Madrid to win = 1/ 3.77= 0.265

But, wait, if we sum 0.478+0.279+0.265=1.022 > 1, and I remember that the sum of all the possible outcomes of a match should be equal to 1…

What is that 0.022?

It is called the vig (or vigorish), juice, margin or overround.

This way, the bookies pay less than they receive through the bets of the users. 

The payout is 1/(1+0.022)=0.9781.

If the bet amounts of each outcome are proportional to the inverse of the odds, the bookies win a “commission” of (1-0.9781)=0.0215=2.15% of the total amount bet.

The fundamentals of value betting are:

→ As the bookies apply this margin to the odds resulting in lower odds than the inverse of the probability, you need to win a little bit more than the probabilities estimated by the bookies if you want to obtain profit.

But, which are the probabilities estimated by the odds?

Following the previous example, the bookie’s estimation of the probabilities are always slightly lower than the inverse of the odds.

That means that Pinnacle’s estimation of the probability of Madrid to win is lower than 0.478, the probability of a draw is lower than 0.29 and the probability of Atletico to win is lower than 0.265.

Ok, but again,

Which are the “true probabilities”, and their inverse, the “true odds”, according to the bookie’s odds?

→ Nobody, except the bookies and their providers, knows exactly how they apply the margin to the true odds.

However, there are several methods to calculate the “true odds”, or “remove the margin”.

Which one the bookies are using is impossible to know.

The methods

The methods to remove the margin we have included in the true odds calculator are:

• Equal margin (EM)
• Margin proportional to odds (MPTO)
• Shin (SHIN)
• Odds ratio (OR)
• Logarithmic (LOG)

Let’s go back to the Real Madrid vs Atlético odds. We are going to calculate the “true odds” and “true probabilities” with the 5 methods by selecting 3 outcomes and writing the odds in the corresponding fields:

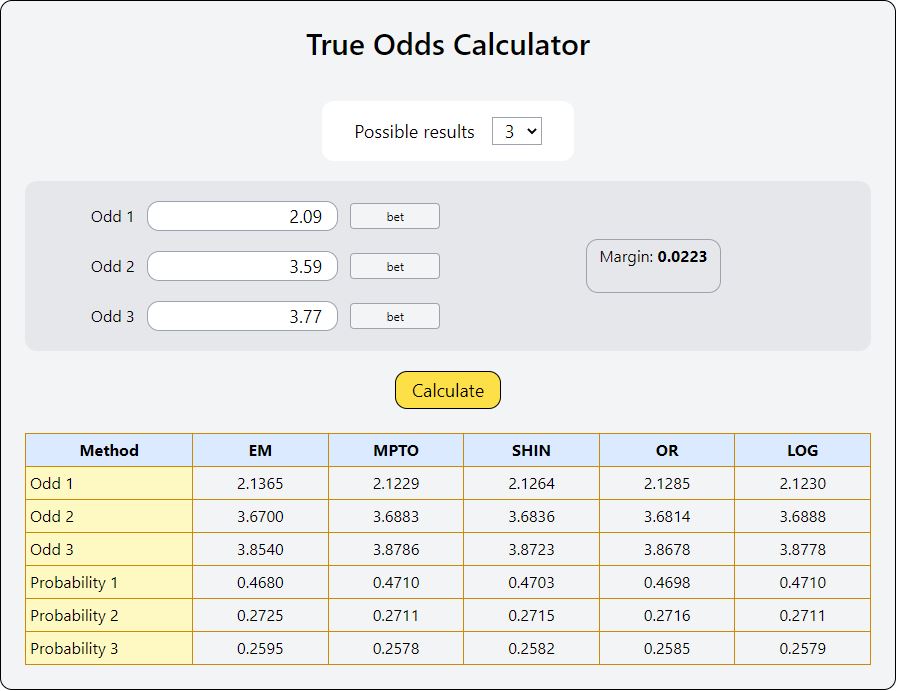
Equal margin

The margin is 0.0223. The “Equal margin” method multiplies the odds by the margin:

This method is very simple, perhaps too much. The bookies probably do not use it to calculate the odds from the probabilities.

Margin proportional to odds

With this method, the higher odds, the more margin is applied. It is calculated as:

This method is a good approximation, better than the EM method, and it is very useful as it is very fast to calculate.

Shin

You can find a detailed explanation of this method in this article by Stephen Clarke et al. In a simply way, it consists on finding the value of “c” that produces a sum of all the probabilities equal to 1:

Don’t ask me why, but if you start with a value of c=0, and iterate values of “c” until the sum of all the probabilities is 1, you have solved the Shin Method. You can find more about the procedure to solve it iteratively in the source code of this blog article.

It results in a very small difference with the MPTO method, but much more difficult to calculate. Is it worth it?

Odds ratio

The odds ratio is explained in detail by Keith Cheung in the following link. It consists also on finding the value of “c” that produces a sum of all the probabilities equal to 1:

In this case, you need to start with a value of c=1, and iterate until the sum of probabilities is equal to 1. You also need some iterations and it can take more time to calculate.

Logarithmic

This method is explained and compared with other methods in this article by Joseph Buchdahl. In this case, the formula used to calculate the probabilities that result in a sum equal to 1 is very simple:

Although it is a very simple formula, you also need to iterate starting with a value of c=1. It is considered to result in very precise results, but as an iterative method, you need more time and write some code to use it.

Why and when do I need to remove the margin?

By removing the margin, you are calculating the estimation of the probabilities by the bookmaker at early or opening odds, or the probabilities obtained by the “wisdom of the crowd” theory at closing odds.

There are several ways to apply this “true odds” or “true probabilities”:

• Find value bets with Positive Expected Value by using sharp bookies odds
• Estimate the Expected Value of a bet by calculating the CLV

Finding value bets

If you consider that the pinnacle odds, a “sharp” bookie, are based on a good estimation of the probabilities, then you can remove the margin and calculate the “true odds” without margin in order to find value bets.

If you find any other bookmaker with odds higher than the “true odds”, you are betting with Positive Expected Value (EV+).

With the example above, if the pinnacle odds are based on a good estimation of the probabilities, and you have a good method to remove the margin, then you have the “true odds” and you can compare them with other bookies to calculate the EV.

If you find, for example, that the odds of a home win are 2.15 in another bookie, as 2.15>2.1230 (Log), then you have a positive Expected Value of: 2.15/2.123-1=0.0127=1.27%.

It is not a very high yield, but if you bet a lot of times in this situation, you will have a profit in the long term. So,

Remember that you need to remove the margin before considering that a difference of odds between bookmakers is enough to find value in the higher odds.

You can use the true odds calculator to estimate this EV very fast:

1. Enter the odds of a sharp bookie, in this case Pinnacle, in the left column
2. Select the odds you are comparing. In this case, for a home win, Odds 1
3. Input the higher odds of the bookmaker you have found in the column at the right
4. Click on the “Calculate” button.

If the final “EV” row shows positive values, then it is worth betting on the higher odds.

Calculating the CLV

If you had bet the day previous to the match on the Real Madrid victory, at odds 2.09, you would have probably bet with positive Expected Value, considering that the closing odds are based on a much better estimation of the true probability of each outcome and they have ended in a much lower value.

In this case, the closing odds of the match a few minutes before the start were:

How can we calculate the EV of betting the day before the match on a home victory at odds 2.09? We can use the true odds calculator too:

1. In this case we use the closing odds in the left column.
2. Then we select the result we have bet (Odds 1, corresponding to the home victory odds)
3. In the field under the margin, we input the odds we have bet
4. Then click on calculate

In the last “EV” row of the table, you can find the long term Expected Value of your bet. According to the Log method, it is 0.0699, a very nice one.

If the last row shows positive values in most of your bets when comparing them with the closing odds, then you are “beating the closing line” and you will probably obtain profit in the long term.

If you do not know what the expected value is, see this article:

What Is Expected Value? EV Calculator For Betting

Be open minded

Here we have shared with you a way to remove the margin and estimate the “true probabilities” according to the odds.

We hope you find it useful and help you to understand the very small edge we usually have when betting, and how important it is to always bet on the higher available odds among many bookmakers.

If you have any example you want to share or you have any question, you can use the comments of this post or write to info@valuebettingblog.com.

But don’t stick to the CLV, these “true probabilities” are a good estimation on average when analysing hundreds of matches. However, they are not always right in an individual match. In my opinion, there are two ways of winning or getting value bets:

1. You know something BEFORE the market includes this information into the odds. Your method is faster or you just don’t need to wait for higher liquidity or higher odds.
2. You have BETTER information than the market at the moment of the bet, and this info can be later included into the odds or not, even if you are placing the bet.

If you usually get value according to 1), you would never win by betting close to the start of the match, and the sooner you bet, the better.

If you get value according to 2), with a different source of information of analysis than the bookies and the wisdom of the crowd, sometimes it is better to wait for higher odds or higher liquidity, and you could even wait to the start of the match, although it is much harder to win by betting at this moment when all the information about the match has been taken into account in the odds.

Note: Remember that you can read and copy the code of this true odds calculator in the source code of this web page. You can also download a Matlab version here.

If you use it, please mention The Value Betting Blog and the authors of the formulas I have used in this article. Thanks!

@Matlab code that removes odds margin according to 6 different methods
1.Equal margin
2.Margin proportional to odds
3.Additive
4.Shin
5.Odds ratio
6.Log function
Thanks to @Gingfacekillah for the R code, and @12Xpert, S. Clarke, H.S. Shin and K. Cheung.https://t.co/IuEQCNUVCa pic.twitter.com/8fs362o1st

— Miguel Figueres (@Miguel_Figueres) February 7, 2019